- This topic has 0 replies, 1 voice, and was last updated 3 năm by
.
CFA2.DER: Meredith Whitney Case Scenario, 0.008396
-
Học viên: em có làm 1 set deri có chỗ không hiểu ạ:
Meredith Whitney Case Scenario
Meredith Whitney is a senior consultant in the Swaps Advisory Group of DCM Capital, an independent advisory firm. Whitney will be preparing to meet with three clients who need advice on structuring and implementing a swap program to manage their interest rate exposures. She is assisted by a junior analyst from the fixed income group, Toni Yang.
For her meetings, Whitney plans to use the data presented in Exhibit 1 below.
EXHIBIT 1CURRENT TERM STRUCTURE OF RATES (%)
Days Libor Euribor Hibor
90 1.42 1.86 1.22
180 1.84 2.11 1.53
270 2.12 2.24 1.7
360 3.42 2.34 1.87
Note: Libor is the London Interbank Offered Rate. Euribor is the Euro Interbank Offered Rate. Hibor is the Hong Kong Interbank Offered Rate. All rates shown are annualized.
Whitney’s first meeting is with Novatel, a US based company that currently has an outstanding loan of $250,000,000 that carries a 5.15% fixed interest rate. Novatel’s managers feel that the current interest rate on the loan is high and they also believe that interest rates are poised to decline. Whitney advises Novatel to enter into a one-year pay-floating Libor receive-fixed interest rate swap with quarterly payments. The notional principal on the swap will be $250,000,000. Whitney’s first task is to determine the appropriate swap rate.
Yang asks Whitney to explain the calculation of the fixed swap rate in a floating-for-fixed interest rate swap. Whitney outlines three possible methods for Yang to consider:
Method 1: The swap rate is the difference between Libor and the fixed interest rate on the bond.
Method 2: The swap rate is the rate that sets the value of the fixed-rate bond equal to the notional principal of the swap.
Method 3: The swap rate is the rate that sets the initial value of the swap equal to zero.
Next, Whitney meets with Grand Manufacturing. This client is based in Hong Kong but requires a €25,000,000 one-year bridge loan to fund operations in Germany. Grand Manufacturing is currently able to borrow euros at an interest rate of 3.75% but wonders if there is a less expensive alternative. Whitney advises Grand to borrow in HK$ and enter into a one-year foreign currency swap with quarterly payments to pay euros at a fixed rate and receive HK$ at a fixed rate. The current exchange rate is HK$11.42 per €1, and the notional amounts will be exchanged at initiation and at maturity.
The final meeting is with KPS Financial Services, a US based asset manager. KPS wants to increase the equity exposure to the US market in one of its portfolios by $100,000,000. Whitney advises KPS to enter into a one-year equity swap with quarterly payments to receive the return on a US stock index and pay a floating Libor interest rate. The current value of the US stock index is 925.
Each client follows Whitney’s advice and immediately implements the recommended position.
Ninety days have passed since Whitney’s initial meetings, and in the interim interest rates have increased dramatically. Whitney’s clients have asked to meet with her to review their positions.
In order to prepare for the meeting, Whitney has obtained updated interest rate data that is presented in Exhibit 2. In addition, she determines that the exchange rate for the Hong Kong dollar is HK$9.96 per €1, and the US stock index is at 905.
EXHIBIT 2TERM STRUCTURE OF RATES 90 DAYS LATER (%)
Days Libor Euribor Hibor
90 2.21 2.94 1.95
180 2.62 3.03 2.45
270 3.73 3.08 2.7
Note: Libor is the London Interbank Offered Rate. Euribor is Euro Interbank Offered Rate. Hibor is the Hong Kong Interbank Offered Rate. All rates shown are annualized.
- Using data in Exhibit 2 and a 30/360 day count, the market value of Novatel’s swap after 90 days is closest to:
A −$602,250.
B −$2,875,000.
C −$2,408,880.
Solution
A is correct. The present value factors for Exhibit 2 are provided below:
PV(90) 0.994505
PV(180) 0.987069
PV(270) 0.972786
For example, PV(180) is calculated as:
11+0.0262×(180/360)=0.987069
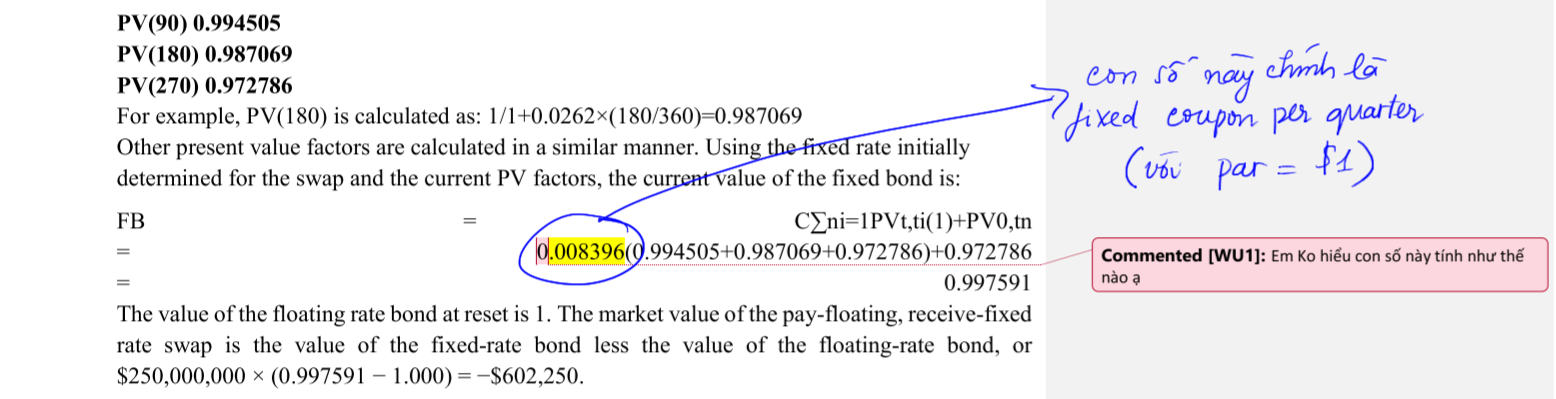
Other present value factors are calculated in a similar manner. Using the fixed rate initially determined for the swap and the current PV factors, the current value of the fixed bond is:
FB = C∑ni=1PVt,ti(1)+PV0,tn
= 0.008396(0.994505+0.987069+0.9
72786)+0.972786
= 0.997591
The value of the floating rate bond at reset is 1. The market value of the pay-floating, receive-fixed rate swap is the value of the fixed-rate bond less the value of the floating-rate bond, or $250,000,000 × (0.997591 − 1.000) = −$602,250.
Using an alternative approach, the new fixed swap rate would be
rFIX = 1.0−PV0,tn(1)∑i=1nPV0,tn(1)
= 1.0−0.9727860.994505+0.987069+0.972786
= 0.009211
And the value of the swap is the difference between the value at the old rate and the value at the new rate, or
V = (FS0−FSt)∑n′i=1PVt,ti
= (0.008396−0.009211)×(0.9945+0.
9871+0.9728)
= −0.002409
The swap value = $250,000,000 × −0.002409 = −$602,250
B and C are incorrect.
Giảng viên: nó ở vị trí của fixed coupon, em tính mãi vẫn không ra hay sao?
Học viên: vâng, con số 0.008396 em ko biết cách tính ạ
Giảng viên: nó chính là pricing swap đấy
18/1/2019
You must be logged in to reply to this topic.
